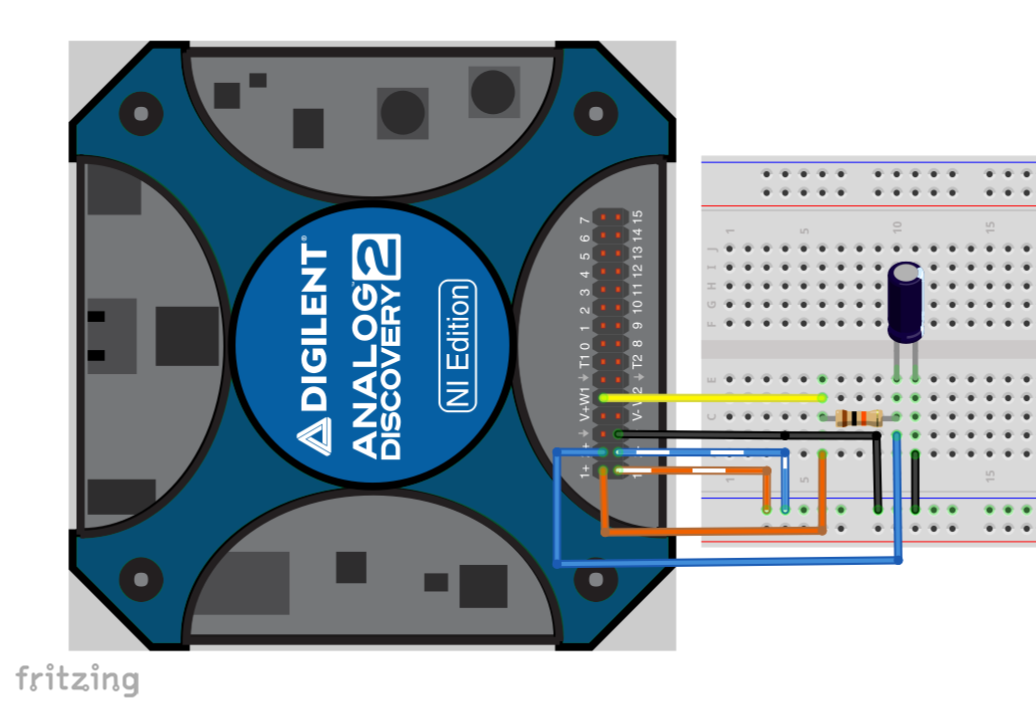
In this chapter, we introduce all fundamental concepts associated with circuit analysis. Electrical circuits are constructed in order to direct the flow of electrons to perform a specific task. In other words, in circuit analysis and design, we are concerned with transferring electrical energy in order to accomplish a desired objective.

In this chapter, we introduce analysis methods based on circuit reduction. Circuit reduction consists of combining resistances in a circuit to a smaller number of resistors, which are (in some sense) equivalent to the original resistive network. Reducing the number of resistors, of course, reduces the number of unknowns in a circuit.

In cases where circuit reduction is not feasible, approaches are still available to reduce the total number of unknowns in the system. Nodal analysis and mesh analysis are two of these.

In this chapter, we introduce the concept of a systems level approach to circuit analysis. In this type of approach, we represent the circuit as a system with some inputs and outputs. We then characterize the system by the mathematical relationship between the system inputs and the system outputs. This relationship is called the input-output relation for the system.

Operational amplifiers (commonly abbreviated as op-amps) are extremely useful electronic devices. Some argue, in fact, that operational amplifiers are the single most useful integrated circuit in analog circuit design. Operational amplifier-based circuits are commonly used for signal conditioning, performing mathematical operations, and buffering.

This chapter begins with an overview of the basic concepts associated with energy storage. This discussion focuses not on electrical systems, but instead introduces the topic qualitatively in the context of systems with which the reader is already familiar. The goal is to provide a basis for the mathematics, which will be introduced subsequently.

First order systems are, by definition, systems whose input-output relationship is a first order differential equation. A first order differential equation contains a first order derivative but no derivative higher than first order - the order of a differential equation is the order of the highest order derivative present in the equation.

Second order systems are, by definition, systems whose input-output relationship is a second order differential equation. A second order differential equation contains a second order derivative but no derivative higher than second order.

In this chapter, we will provide a very brief introduction to the topic of state variable modeling. The brief presentation provided here is intended simply to introduce the reader to the basic concepts of state variable models, since they are a natural - and relatively painless - extension of the analysis approach we have used in Chapters 7 and 8.

In this chapter we will study dynamic systems which are subjected to sinusoidal forcing functions.

In this chapter we discuss representation of signals in terms of their frequency content. We will also represent the frequency content of the input and output signals and the frequency response of the system in graphical format. This leads us to think in terms of using a system to create a signal with a desired frequency content - this process is called filtering.

In this chapter we will address the issue of power transmission via sinusoidal (or AC) signals. This topic is extremely important, since the vast majority of power transmission in the world is performed using AC voltages and currents.